Abstract
We describe the use of thermal models to determine maximum safe power ratings for Faraday rotation isolators. Models are constructed for two distinct isolator topologies. The first model is based on the topology used for legacy isolators. These are the isolators that have been in common usage for more than 40 years and marketed by other vendors. The second model is based on the topology of the isolators produced by Micro Harmonics Corporation (MHC). The primary difference is that the MHC isolators employ a CVD diamond disc for increased power handling while the legacy isolators do not.
AutoDesk NASTRAN is used for the thermal simulations. NASTRAN uses a finite element analysis (FEA) to determine the temperature distribution in arbitrary 3-D objects. The simulator yields a detailed 3-D map of the temperature inside the isolator. Legacy isolators have well established maximum power ratings. In the NASTRAN model for the legacy isolator, the thermal source is set to a value equivalent to the established maximum power rating. The simulation yields the maximum temperature in the legacy isolator when driven at the maximum rated power level. This temperature provides a baseline to determine the maximum power for the MHC isolator.
Introduction
Isolators are used to mitigate standing waves between components by allowing an RF signal to pass in the forward direction but absorbing any signal traveling in the reverse direction. The signals travelling in the reverse direction are absorbed in a resistive element and converted to heat energy. Catastrophic failure can occur due to excessive heating. The isolator power rating is a function of how efficiently the heat can be channeled away from the resistive element. At millimeter-wave frequencies, the primary isolator technology is the Faraday rotation type first described by Barnes [1]. At the heart of this isolator are a pair of alumina cones and a cylindrical ferrite rod suspended between two rectangular waveguides. The cones are used to couple the primary TE10 propagating mode in the rectangular waveguides to the HE11 mode in the ferrite rod.
 |
| Figure 1 – Left: HFSS simulation showing the surface loss in the input side cone resistive layer. Right: Geometry of uniform heat source used in the NASTRAN thermal simulations. |
The cones are bisected by a resistive layer along the central axis. The resistive layer in the input side cone absorbs most of the power in the reverse travelling signal and converts it to heat energy. The input side resistive layer is used as a heat source in the thermal models. The heat is primarily generated in the area near the cone base as indicated by the HFSS simulation shown in the left side in Figure 1. The simulation shows surface loss in the resistive layer. As a first order approximation, the heat source is modeled as uniform in a subsection of the resistive layer near the base of the cone as shown the graphic in the right side of Figure 1.
The size of the cones and the diameter of the ferrite decrease proportionally as the frequency increases. The length of the ferrite rod does not scale with frequency. In the NASTRAN thermal simulator, the thickness of the resistive layer is modeled at approximately 0.001 inch which is thicker than the actual resistive layer. This is necessary due to difficulties in modelling relatively thin layers in FEA. The thick layer has a minimal impact on the accuracy of the models.
Thermal Modeling of Legacy Isolators
 |
| Figure 2 – Sketch showing the core of a legacy millimeter-wave Faraday rotation isolator. |
In most commercial millimeter-wave Faraday rotation isolators, the ferrite rod and cones are suspended in the rectangular waveguides by a pair of washer shaped supports as shown in Figure 2. We refer to these as legacy isolators.
The cones and ferrite rod are epoxied together and then inserted through the inner holes in the supports. The ferrite/cone assembly is then epoxied to the supports. The support material is typically BOPET, polyimide, mica, styrene, a resin or some other material with a low dielectric constant and low loss at millimeter wave frequencies. Materials with these characteristics are generally in the class of thermal insulators and thus the cones and ferrite are thermally isolated from the metal block. Table 1 shows the dielectric constant and thermal conductivity for some dielectric materials and metals.
| Table 1 – Thermal conductivity and dielectric constant of select materials. | |||
|---|---|---|---|
| Material | Description or Trade Name |
Thermal Conductivity W/(m•K) |
Dielectric Constant |
| Vacuum | 0 | 1 | |
| Polystyrene | 0.146 | 2.53 | |
| BOPET | Mylar®, Duralar® | 0.155 | 3.3 |
| Polyimide | Kapton®, Upilex-S® | 0.2 | 3.5 |
| PTFE | Teflon® | 0.25 | 2.1 |
| Mica | 0.7 | 6.5 | |
| Resin | Stycast 1266® | 0.73 | 3 |
| Borsilicate Glass | Pyrex® | 1.1 | 4.6 |
| Stainless Steel | 15 | ||
| Alumina | 35 | 9.8 | |
| Platinum | 70 | ||
| Silicon | 150 | 11.9 | |
| Aluminum | 237 | ||
| Copper | 400 | ||
| Diamond | High-grade CVD | >2000 | 5.7 |
| Diamond | Crystalline | 2200 | 5.7 |
| Material properties vary by manufacturer, frequency, temperature, etc. | |||
In the legacy isolators, very little heat energy can be channeled away from the cone by thermal conduction through the thermally insulating supports. Rather, the heat is dissipated by means of convection to the surrounding air. The resistive layers are subject to high heat levels and even damage if too much reverse power is incident on the device. Historically this was not an issue as there was very little power available at these frequencies. But as higher power sources are becoming available there is a renewed interest in the power ratings of these devices.
 |
| Figure 3 – Cutaway view of the thermal model for a WR-10 legacy isolator. The two support structures are made from mica and have a washer shape. |
Figure 3 shows a split view (cutaway view) of the thermal model used for the legacy isolator. The cones, ferrite rod, and two washer shaped supports are visible in the sketch.
Mica was used as the material for the two supports in the legacy thermal model. Mica is in the class of thermal insulators with the dielectric constant and thermal conductivity provided in Table 1. Convection boundaries are defined at all exposed surfaces of the cones, ferrite, and the mica supports. Convection boundaries are also defined along the outer periphery of the metal waveguide block. The ambient air temperature in the simulation is 20°C (68°F). Most vendors rate their WR-10 legacy isolators at 1 W maximum reverse power. A single heat source is used in the input cone of the model with the heat equivalent to 1 W of absorbed power.
A NASTRAN simulation result for the WR-10 legacy isolator is shown in Figure 4. The maximum temperature in the simulation is 49°C (120°F) in the input side cone. The temperature is fairly uniform throughout the volume of the input side cone. Thermal gradients are evident in the ferrite and in the mica support washer indicating that there is very little heat conduction in these materials. The temperature of the metal block is nearly uniform at 20 °C because the block is thermally isolated from the heat source. The output cone (right side) temperature is also fairly uniform at 35°C (95°F). Temperatures in the ferrite range from 49°C at the mating surface to the input cone to about 35°C at the mating surface to the output cone.
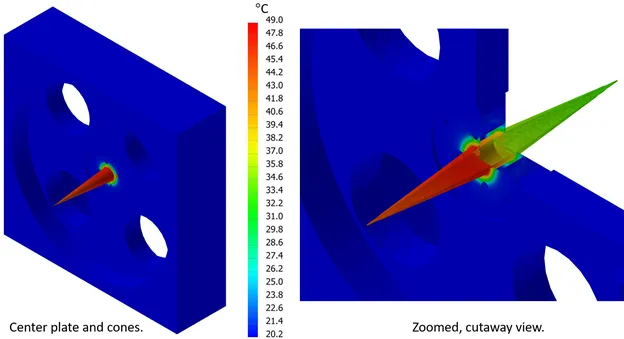 |
| Figure 4 –Thermal model for a legacy WR-10 isolator. The left side graphic shows the entire center plate with the input side cone shown extending outward from the center. The right side graphic shows a zoomed view with a quarter section of the model cut away to expose both cones and the ferrite rod. The maximum temperature is 49°C (120°F). |
The 49°C (120°F) maximum temperature is used as a baseline to determine maximum safe power levels for the Micro Harmonics isolators. The absorbed signal power that gives rise to a maximum temperature of 49°C is deemed the maximum safe power for the MHC isolator.
The 49°C maximum temperature should be considered a best case scenario. The convection boundaries assume that an infinite supply of air at 20°C is available to carry heat away from the cone. However, the cone is contained within a constricted air space (the interior of a WR-10 rectangular waveguide). Therefore, the ambient air temperature will rise over time, much like an oven, leading to higher cone temperatures. The model is useful for setting a safe baseline temperature. The model also clearly demonstrates that the cones and ferrite are thermally isolated from the block temperature.
Thermal Modeling of Micro Harmonics Isolators
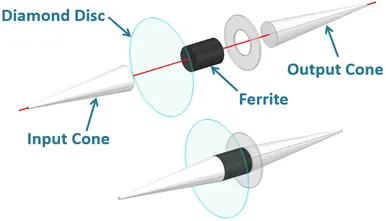 |
| Figure 5 – Sketch showing the location of the diamond disc in Micro Harmonics isolators. |
Micro Harmonics isolators employ a diamond support disc that channels heat from the resistive layer in the cone to the metal waveguide block and thus they can handle greater reverse power levels. No other commercial isolators offer this advantage. The diamond disc is optical-grade CVD. Diamond is the ultimate thermal conductor approaching 2200 W/mK, more than five times higher than copper. The diamond disc is sandwiched between the base of the input cone and the ferrite as shown in Figure 5. The diamond disc is in intimate contact over the entire area of the cone base. This is the optimal location for the diamond disc since it is subject to the highest heat levels.
 |
| Figure 6 – Cutaway view of the thermal model for the Micro Harmonics WR-10 isolator. Two support structures are used. A thermally conductive diamond disc is used on the left side and a thermally insulating BOPET washer is used on the right side. |
A cutaway view of our WR-10 thermal model is shown in Figure 6. A single heat sourced is defined near the base of the input side (left side) ceramic cone. The heat source is similar to the one shown in Figure 1. The diamond support disc on the left side is sandwiched between the ferrite rod and ceramic cone, extends beyond the absorber, and overlaps the aluminum block on the outer periphery. The diamond provides a thermal conduction path from the ceramic cone to the aluminum block. The right-side support is washer shaped and made of BOPET (Mylar™). BOPET is a thermally insulating material. The dielectric constants and thermal conductivities of BOPET and diamond are given in Table 1.
In the isolator, most of the heat energy from the absorbed reverse travelling signal is generated in the region near the base of the ceramic cone on the left side. The left side cone is the one that is attached to the thermally conducting diamond disc. A small fraction of the reverse travelling signal is reflected at the left side cone, propagates (and rotates) back through the ferrite, and is absorbed in the resistive layer in the right side cone.
 |
| Figure 7 – Heat dissipation via CVD diamond in a Micro Harmonics isolator. |
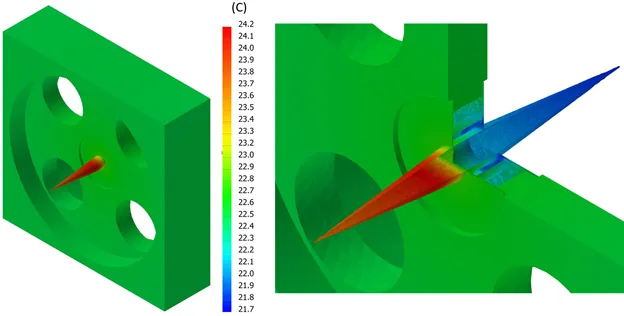 |
| Figure 8 –Thermal model for a Micro Harmonics WR-10 isolator. The left side graphic shows the entire center plate with the input side cone shown extending outward from the center. The right side graphic shows a zoomed view with a quarter section of the model cut away to expose both cones and the ferrite rod. |
Figure 7 shows a cutaway view of the MHC isolator with the core assembly sitting in the metal block. The cones and ferrite suspended in the waveguides are visible. The material marked “absorber” is used to suppress higher-order modes in the region near the ferrite. None of the reverse power is dissipated in the absorber but rather in the resistive layer in the input cone.
The diamond disc is epoxied to the metal waveguide block over its entire periphery and thus provides an excellent conduit to channel heat away from the resistive layer in the input cone. The red arrows indicate the path of heat flow. Even at low reverse power levels, our isolators consistently run cooler with reduced thermal stress on the epoxy joints.
A NASTRAN simulation result for the Micro Harmonics (MHC) isolator is shown in Figure 8. In this simulation, the metal center plate is not tied to a thermal ground but rather convection boundaries are used on the four outer most surfaces. A diamond disc is used on the left side and a BOPET support washer is used on the right side. Convection boundaries are applied to all surfaces of the cone, ferrite rod and BOPET support washer. The ambient air temperature in the simulation is 20°C. A single heat source is used in the input side cone (left side) with the heat equivalent to 1 W of dissipated power.
The maximum temperature is 24 °C in the input side cone. That temperature is fairly homogeneous throughout the volume of the input side cone except near the base where a thermal gradient is evident. The metal block temperature is nearly uniform at 22.7 °C. The output side cone temperature is also fairly homogeneous at 21.8 °C. Temperatures in the ferrite range from 21.8°C – 22.7°C.
No thermal gradient is apparent in the diamond disc. Diamond is an excellent heat conductor and acts as a thermal short circuit between the base of the cone and the metal waveguide block. The block has a relatively uniform temperature near 23°C (73°F). Normally, the block would be attached to larger outer metal plates and other connected components. Rather than using convection boundaries on the outer edges of the block it might be better to impose a thermal ground at 20°C along the periphery which would lower the maximum temperature by another one or two degrees.
Analysis of the Simulation Data
Both models use a WR-10 geometry, 1 W absorbed power, and 20°C ambient air temperature with convection boundaries. The legacy isolator model had a maximum temperature of 49°C. The MHC model with the diamond disc had a maximum temperature of 24°C which is only 4° above ambient. Clearly the diamond does a great job of transferring heat away from the resistive layer in the cones.
 |
| Figure 9 – Simulation of maximum temperature -vs- absorbed RF power for WR-10 isolators. |
In Figure 9 we show the maximum temperature in the isolator as a function of the absorbed RF power for the two WR-10 models. The simulations indicate that the relationship between power and temperature is linear. This makes sense since we do not include any temperature dependence of the materials in the models. The slopes of the two curves are vastly different. The models indicate that our WR-10 isolator should be able to safely handle a reverse power of 7 W rather than the industry standard of 1 W. But the Micro Harmonics advantage is not limited to operation at very high power levels. At any given power level, the Micro Harmonics isolator operates at a much lower temperature. This translates into increased meantime to failure and improved reliability.
Power Ratings Advertised by Other Vendors
Power ratings advertised by six other vendors are shown in Figure 10. The vendors are designated as V1 (vendor # 1) through V6. A logarithmic curve fit to the data is shown. The x-axis is the EIA waveguide flange designation. For instance, WR-10 is the number 10 on the x-axis.
 |
| Figure 10 – Maximum isolator power ratings advertised by six vendors. The red line (CF) is a logarithmic curve fit to the data. WR-XX is the EIA waveguide flange designation (eg. WR-10). |
The data indicate that the power ratings are segmented rather than following a smooth curve. Four of the six vendors have their WR-10, WR-12, and WR-15 isolators rated at 1 W with no change in the power rating corresponding to changes in the physical sizes of the cones and ferrite. The ratings are somewhat arbitrary. The goal is to keep the reverse power levels low enough so that the isolator does not incur any significant damage from heat over time.
Many electronic components have a temperature dependent average lifetime. The most likely failure point in the isolator is the thin resistive film used to absorb the reverse travelling signal. This is also the hottest spot in the isolator. A common rule of thumb is that every 10°C increase in temperature reduces component life by half. The rule is based on applying the Arrhenius equation relating the rate of chemical reactions to failure mechanisms that occur in electronics. The rule of thumb is simplified but the general principle is sound. It is always advantageous to run components at lower temperatures. At a given reverse power level, a Micro Harmonics isolator operates at a much lower temperature than a traditional style isolator. This translates into higher reliability and longer meantime to failure.
Power Rating Summary
Table 2 shows a summary of the power ratings for millimeter-wave isolators. The power ratings in the column marked “Legacy Isolators” are based on advertised specifications from other vendors. The power ratings for the Micro Harmonics isolators are based on the thermal models described here. Data will be added as it becomes available.
| Table 2 – Power ratings for millimeter-wave isolators. | ||||
|---|---|---|---|---|
| EIA Flange Designation |
Frequency Band (GHz) |
Legacy Isolators Pmax (W) |
Micro Harmonics Rated Pmax (W) |
|
| WR-28 | 26 – 40 | 2.5 | 4.0 | |
| WR-19 | 40 – 60 | 1.5 | 3.4 | |
| WR-15 | 50 – 75 | 1.5 | 3.0 | |
| WR-12 | 60 – 90 | 1 | 2.7 | |
| WR-10 | 75 – 110 | 1 | 2.3 | |
| WR-9* | 82 – 123 | 2.1 | ||
| WR-8 | 90 – 140 | 1 | 1.8 | |
| WR-6.5 | 110 – 170 | 0.75 | 1.5 | |
| WR-5.1 | 140 – 220 | 0.75 | 1.0 | |
| WR-4.3 | 170 – 260 | 0.1 | 0.7 | |
| WR-3.4 | 220 – 330 | 0.1 | 0.4 | |
| WR-2.8 | 260 – 400 | 0.3 | ||
| * WR-9 is a non-standard waveguide band. | ||||
Conclusion
The data presented here should be regarded as preliminary in nature. The thermal models continue to be refined. But there are a few observations that can be made. The models clearly show that the diamond discs used in the Micro Harmonics isolators are effective at transporting heat from the resistive layer in the cone to the metal waveguide block. The heat transfer significantly lowers the maximum temperature in the cone. The Micro Harmonics isolators are therefore able to handle significantly higher power levels. At any given power level, the Micro Harmonics isolators run at lower temperatures which translates into higher reliability and longer component lifetime. For now, we continue to rate our isolator maximum power at levels similar to those used in the industry. But once our thermal studies have been completed, the ratings will be revised upward accordingly.
References
[1] C.E. Barnes, “Broad-band Isolators and Variable Attenuators for Millimeter Wavelengths,” IEEE Trans. Microwave Theory Tech., vol. 9, pp. 519-523, 1961.
